|
|
[MODERATOR]
★ FUN WITH MATH : [Setiap Hari Isnin]★
[Copy link]
|
|
|
maaf sebab lambat...
mggu ni tersngt le sibuk...  |
|
|
|
|
|
|
|
|
|
|
|
Reply 3# mnm77
terima kasih & tahniah kerana menjawab...  |
|
|
|
|
|
|
|
|
|
|
|
Reply 8# lavender_aqua
terima kasih & tahniah kerana menjawab...  |
|
|
|
|
|
|
|
|
|
|
|
Reply 201# midory
Salam....
Just my opinion.
Bagi saya, tahap soalan tu (soalan 10) tidaklah senang. Kena ada ilmu yang cukup pasal complementary function & particular solution, baru boleh buat soalan tu. Tambahan, untuk soalan tu, particular solution tu SPECIAL CASE, tak boleh guna cara kebiasaan.
Untuk selesaikan soalan tu, mesti tahu special case. Kalau tak tahu, mustahil boleh dapat jawapannya. Kedua-dua cara yang saya tunjukkan tu dua-dua special case.
1. Undetermined Coefficient: Special Case: Multiply independent variable (x) to the term already existed in the complementary function. Otherwise impossible to solve!
2. Operator D: Multiply independent variable (x) to the term that causing 'divide by zero' AND differentiate the denominator of the operator function. Otherwise impossible to solve!
Cara ketiga, variation of paramater saya belum sempat pastikan jawapannya kerana jalannya lebih panjang.
Just to share knowledge.... |
Rate
-
1
View Rating Log
-
|
|
|
|
|
|
|
|
|
|
|
Post Last Edit by mnm77 at 10-4-2012 08:25
Reply 186# dauswq
Just sharing again....
Soalan 9, jawapan am bukannya dalam bentuk y₁ dan y₂.
y₁ dan y₂ adalah punca-punca (roots) penyelesaian kepada ODE tersebut, belum lagi dalam bentuk umum.
Punca-punca tersebut bergantung kepada punca persamaan kuadratik (auxiliary/characteristic equation)
Roots of the auxiliary equation (m₁ & m₂) can be EITHER:
1. Two Real and distinct roots: b² - 4ac > 0
Complementary function:
=======> y = A eᵐ¹ˣ + B eᵐ²ˣ
(A & B are arbitrary constants)
2. Real and repeated roots: b² - 4ac = 0
- or two same roots or double roots (m = m₁ = m₂)
Complementary function:
=======> y = A eᵐˣ + Bx eᵐˣ
3 Complex roots: b² - 4ac < 0
Complementary function:
=======> y = A eᵖˣ cos(qx) + B eᵖˣ sin(qx)
where the complex roots are:
m = p±qi
i =√(-1)
Jawapan am untuk soalan 9 sepatutnya: y = A exp(-5x) + B exp(x) ====> Case 1 di atas
Kalau saya salah, sama-sama kita bincang dan betulkan, supaya ilmu yang disampaikan itu bermanfaat 
WAllahu a'lam |
|
|
|
|
|
|
|
|
|
|
|
Reply 205# midory
Masih tak jelas, kalau midory/daus, atau sesiapa yang faham penjelasan tu tolong explain step by step, really appreciate.
Thanks |
|
|
|
|
|
|
|
|
|
|
|
1. Undetermined Coefficient: Special Case: Multiply independent variable (x) to the term already existed in the complementary function. Otherwise impossible to solve!
2. Operator D: Multiply independent variable (x) to the term that causing 'divide by zero' AND differentiate the denominator of the operator function. Otherwise impossible to solve!
Cara ketiga, variation of paramater saya belum sempat pastikan jawapannya kerana jalannya lebih panjang.
Just to share knowledge....
mnm77 Post at 9-4-2012 08:59 
Salam...
Ok just sharing knowledge, hopefully beneficial for those who interested in calculus and for solving 2nd Order ODE.
Soalan 10, penyelesaian melalui kaedah 'Variation of Parameter'
Variation of Parameter
===============
Please note that the formula of variation of parameter is not discussed here. Otherwise it will become quite lengthy!
First, we need to obtain the complementary function, (which is shown in the previous method: Undetermined Coefficient, so not repeated here)
Then....

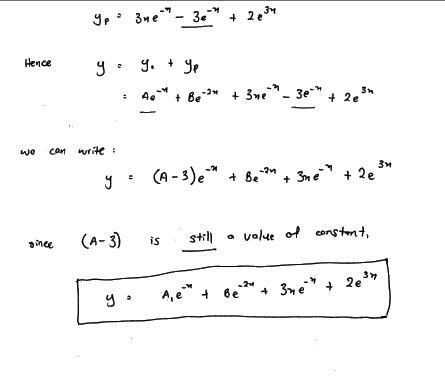
NOTICE:
Using this method, the particular solution obtained IS DIFFERENT to that obtained from Method of Undetermined Coefficient and Operator D Method. However, the general solution is the SAME,
due to the constant term in "3 exp(-x)", which is 3,
can be factorised together with the constant A from the term "A exp(-x)" coming from complementary function.
I think this example (question) is VERY USEFUL for those who want to know and to learn how to solve Non-Homogeneous Second Order Linear Ordinary Differential Equation.
WAllahu a'lam |
Rate
-
1
View Rating Log
-
|
|
|
|
|
|
|
|
|
|
|
Post Last Edit by mnm77 at 13-4-2012 17:47
Tips for solving ODE, must first determine TYPE of ODE. Then utilise appropriate method.
2 tahun lepas mmg giat bg soalan math smpi diorg pening kepala kerana soalan2nya adalah dr peringkat uni...lepas tu makin suram org tak mau jwb
dauswq Post at 28-2-2012 16:30 
Soalan calculus yang diberi memang peringkat uni, jadi secara wajarnya saya rasa memang ramai yang tak boleh jawab, melainkan jika ada belajar ODE & calculus tahap universiti, dan faham....
(ramai yang belajar ODE & CALCULUS tapi masih susah faham, tambah pula yang mengajar pun sebenarnya asasnya tak berapa kukuh, maka pengajaran pun jadi susah kepada students)
Contoh asas yang mudah tapi masih ramai yang keliru: apa beza linear & non-linear ODE? Siapa boleh terangkan? |
|
|
|
|
|
|
|
|
|
|
|
For differential equation with independent variable x:
1) y" + 3y' + 2y = x² + cos x
2) y'' + 3y' + 2y = 5x + cos y
Yang mana linear ODE dan yang mana non-linear ODE?
Oklaa... if I'm being too serius, nanti orang takut nak jawab pulak....
jawapannya:
1) y" + 3y' + 2y = x² + cos x (LINEAR ODE)
2) y'' + 3y' + 2y = 5x + cos y (NON-LINEAR)
Kalau boleh bagitau sebab, maknanya dah faham konsep linearity of ODE. |
|
|
|
|
|
|
|
|
|
|
|
Tips for solving ODE, must first determine TYPE of ODE. Then utilise appropriate method.
Soal ...
mnm77 Post at 13-4-2012 17:40 
linear ODE adalah yang kuasa 2...betul tak??  |
|
|
|
|
|
|
|
|
|
|
|
linear ODE adalah yang kuasa 2...betul tak??
kelapamuda Post at 15-4-2012 21:11 
Ye betul.... boleh jelaskan kenapa? Hanya sekadar perkongsian... |
|
|
|
|
|
|
|
|
|
|
|
Ye betul.... boleh jelaskan kenapa? Hanya sekadar perkongsian...
mnm77 Post at 16-4-2012 09:05 
sbb bile plot..ianya membentuk garis lurus...ke? huwaaaaa....tak tau... ..kalo yg non-linear...have several point of *puncak?*..concave up or concave down... ..kalo yg non-linear...have several point of *puncak?*..concave up or concave down...
kat dlm soalan yg dorang bg ni..mostly is calculus...but r they know the application? kalo tnye application of calculus, most people ckp...area, volume...
tapi bg aku, calculus is not about area or volume, but application in sense..hehehe...teruk tak aku? sense sbb dia melatih tuk kita jadi lebih alert... |
|
|
|
|
|
|
|
|
|
|
|
Post Last Edit by mnm77 at 16-4-2012 12:24
sbb bile plot..ianya membentuk garis lurus...ke? huwaaaaa....tak tau.....kalo yg non-linear...have several point of *puncak?*..concave up or concave down...
kelapamuda Post at 16-4-2012 09:47 
Aitt tadi kata yang ada kuasa 2 tu linear.... plot x kuasa 2 tu garis lurus ke?
Nampak macam dah keliru ni...
Takpe... kayuh pelan-pelan..... ODE ni kena faham basics dia betul-betul....
Lesson 1: Linearity of Algebraic Equation
Sebelum kita masuk linearity of ODE, kita kena faham konsep linear.
For algebraic equation, linear equation indicates function of straight line, in other words, the power of x is 1 (or degree 1).
Example y = mx + c (equation of straight line, linear equation)
If power of x is not 1, then the algebraic equation is NON-LINEAR
Example:
y = x²
y = 1/x = x^(-1)
Lesson 2: Linearity of ODE
1. First understand dependent and independent variable
Example:
dy/dx + y = 0
y is dependent variable
x is independent variable
x'' + 3x' + x = t² +t
x is dependent variable
t is independent variable
2. Understand order of ODE so that not to confuse with degree of ODE
- the highest derivative in ODE is the order of that ODE
x'' + 3x' + x = t² +t (2nd order)
dy/dx + y = 0 (1st order)
y''' + y = 0 (3rd order)
d⁶y/dx⁶ + y = 0 (6th order)
3. Understand degree of ODE
- the highest power of the highest derivative
dy/dx + y = 0 (1st degree)..... (1st order)
dy/dx + y² = 0 (1st degree)..... (1st order)
(dy/dx)² + y = 0 (2nd degree)...... (1st order)
d²y/dx² + y⁶ = 0 (1st degree) ...... (2nd order)
(d⁴y/dx⁴)² + d⁴y/dx⁴ + (d²y/dx²)⁶ + y = 0 (2nd degree) ...... (4th order)
Then we are ready to understand what is LINEAR ODE and NON-LINEAR ODE
Actually it is not very difficult, but if we don't understand the above basics in ODE, then we might always be confusing ourselves.... |
Rate
-
1
View Rating Log
-
|
|
|
|
|
|
|
|
|
|
|
kat dlm soalan yg dorang bg ni..mostly is calculus...but r they know the application? kalo tnye application of calculus, most people ckp...area, volume...
tapi bg aku, calculus is not about area or volume, but application in sense..hehehe...teruk tak aku? sense sbb dia melatih tuk kita jadi lebih alert...
kelapamuda Post at 16-4-2012 09:47 
Application of ODE sangat banyak. Area & Volume tu lebih kepada integration....(masih dalam calculus)
Nak kira/anggar kadar tindakbalas kimia, guna ODE
Nak kira/anggar perubahan suhu sesuatu benda yang dipanaskan, guna ODE
Nak kira masa yang diambil untuk memberhentikan sebuah kenderaan bila tekan brek, guna ODE
Pendek kata, apa-apa yang ada kaitan perubahan (rate of change), boleh diformulakan dalam bentuk ODE |
|
|
|
|
|
|
|
|
|
|
|
Post Last Edit by mnm77 at 16-4-2012 17:56
continue...
Lesson 2: Linearity of ODE
4. ODE is linear if:
- The degree of ODE is 1 with respect to dependent variable as well as to any of its derivatives (meaning the power of dependent variable as well as the derivatives are all equivalent to 1),
- There should be no product of dependent variable with its derivatives.
- The non-linearity of ODE does not depend on the degree of independent variable (x).
(1) y" + 3y' + 2y = x² + cos x (LINEAR ODE)
(2) y'' + 3y' + 2y = 5x + cos y (NON-LINEAR ODE)
Jadi boleh jelaskan sebab kenapa no (1) linear ODE dan no (2) non-linear ODE?
1) Power of x is 2, but that does not count for non-linearity of ODE because x is independent variable.
cos x is non-linear in x (independent variable), also does not count for non-linearity of ODE
2) Here, cos y is non-linear in y (dependent variable). Hence ODE is non-linear, since power of y is not 1 anymore (degree is not 1)
Note: cosine function is non-linear.
Also cos y can be expressed as power series of y
http://en.wikipedia.org/wiki/Taylor_series |
Rate
-
1
View Rating Log
-
|
|
|
|
|
|
|
|
|
| |
Category: Belia & Informasi
|